To set up integrals in polar coordinates, we had to understand the shape and area of a typical small region into which the region of integration was divided. We need to do the same thing here, for three dimensional regions. The Cartesian coordinate system provides a straightforward way to describe the location of points in space. Some surfaces, however, can be difficult to model with equations based on the Cartesian system. In this section, we look at two different ways of describing the location of points in space, both of them based on extensions of polar coordinates.
Similarly, spherical coordinates are useful for dealing with problems involving spheres, such as finding the volume of domed structures. The crux of setting up a triple integral in spherical coordinates is appropriately describing the "small amount of volume," dV, used in the integral. However, the usefulness of these coordinate systems does not lie in the variety of surfaces that they can describe nor the regions in space these surfaces may enclose. Rather, cylindrical coordinates are mostly used to describe cylinders and spherical coordinates are mostly used to describe spheres. These shapes are of special interest in the sciences, especially in physics, and computations on/inside these shapes is difficult using rectangular coordinates. Set up the triple integrals that find the volume of this region using rectangular, cylindrical and spherical coordinates, then comment on which of the three appears easiest to evaluate.
A similar situation occurs with triple integrals, but here we need to distinguish between cylindrical symmetry and spherical symmetry. In this section we convert triple integrals in rectangular coordinates into a triple integral in either cylindrical or spherical coordinates. Triple integrals can often be more readily evaluated by using cylindrical coordinates instead of rectangular coordinates. Some common equations of surfaces in rectangular coordinates along with corresponding equations in cylindrical coordinates are listed in . These equations will become handy as we proceed with solving problems using triple integrals.
Some common equations of surfaces in rectangular coordinates along with corresponding equations in cylindrical coordinates are listed in Table \(\PageIndex\). By changing the display options, we can see that the basis vectors are tangent to the corresponding coordinate lines. Changing $\theta$ moves $P$ along the $\theta$ coordinate line in the direction $\hat_\theta$, and similarly for the other coordinates.
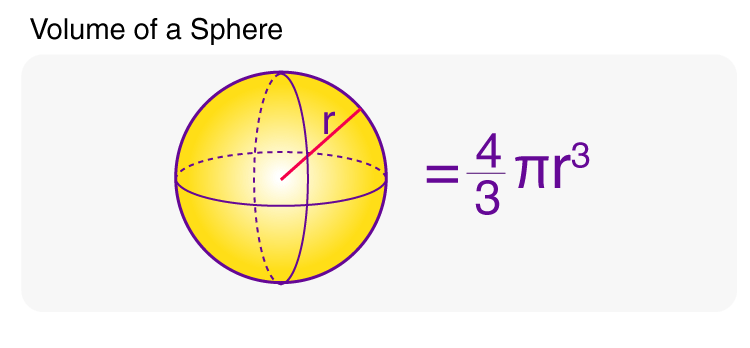
Let D be the region in space bounded by the sphere, centered at the origin, of radius r. Use a triple integral in spherical coordinates to find the volume V of D. As stated before, spherical coordinate systems work well for solids that are symmetric around a point, such as spheres and cones. Let us look at some examples before we consider triple integrals in spherical coordinates on general spherical regions.
In the spherical coordinate system, we again use an ordered triple to describe the location of a point in space. In this case, the triple describes one distance and two angles. Spherical coordinates make it simple to describe a sphere, just as cylindrical coordinates make it easy to describe a cylinder. Grid lines for spherical coordinates are based on angle measures, like those for polar coordinates. Also recall the chapter prelude, which showed the opera house l'Hemisphèric in Valencia, Spain. It has four sections with one of the sections being a theater in a five-story-high sphere under an oval roof as long as a football field.
Inside is an IMAX screen that changes the sphere into a planetarium with a sky full of \(9000\) twinkling stars. Using triple integrals in spherical coordinates, we can find the volumes of different geometric shapes like these. That is located in the first octant as triple integrals in cylindrical coordinates and spherical coordinates, respectively. As triple integrals in cylindrical coordinates and spherical coordinates, respectively. There are many different conventions for spherical coordinates notation, so it's important to check which variant is being used in any document. The convention used here is common in mathematics.
In physics it is also common to use the same angles, but to reverse the symbol convention so that $\phi$ is the azimuth and $\theta$ is the inclination. Many different names for the coordinates are also used, with the inclination also being called the zenith angle, polar angle, or normal angle. Alternatively, the inclination may be replaced by the elevationmeasured up from the $x$-$y$ plane.
In mathematics and physics, spherical polar coordinates form a coordinate system for the three-dimensional real space . Three numbers, two angles and a length specify any point in . The two angles specify the position on the surface of a sphere and the length gives the radius of the sphere. The concept of triple integration in spherical coordinates can be extended to integration over a general solid, using the projections onto the coordinate planes.
Note that \(dV\) and \(dA\) mean the increments in volume and area, respectively. The variables \(V\) and \(A\) are used as the variables for integration to express the integrals. Cylindrical coordinate systems work well for solids that are symmetric around an axis, such as cylinders and cones. Let us look at some examples before we define the triple integral in cylindrical coordinates on general cylindrical regions. We can use triple integrals and spherical coordinates to solve for the volume of a solid sphere. This gives us a generalized method, which can be easily extended to triple integrals, that makes it easier to work in different coordinate systems such as spherical coordinates.
Spherical polar coordinates are useful in cases where there is spherical symmetry, in interactions or in boundary conditions . In such cases spherical polar coordinates often allow the separation of variables simplifying the solution of partial differential equations and the evaluation of three-dimensional integrals. 24., a triple integral in spherical coordinates is given. Describe the region in space defined by the bounds of the integral.
Again, this development of dV should sound reasonable, and the following theorem states it is the appropriate manner by which triple integrals are to be evaluated in spherical coordinates. The green dot is the projection of the point in the $xy$-plane. You can visualize each of the spherical coordinates by the geometric structures that are colored corresponding to the slider colors. The length of the red line segment from the origin is $\rho$. The angle of the green portion of the disk in the $xy$-plane is $\theta$.
The angle of the blue portion of the vertical disk is $\phi$. You can also move the large red point and the green projection of that point directly with the mouse. Spherical coordinates can be a little challenging to understand at first. Spherical coordinates determine the position of a point in three-dimensional space based on the distance $\rho$ from the origin and two angles $\theta$ and $\phi$. If one is familiar with polar coordinates, then the angle $\theta$ isn't too difficult to understand as it is essentially the same as the angle $\theta$ from polar coordinates. But some people have trouble grasping what the angle $\phi$ is all about.
First of all, you may ask why I used cartesian coordinates to compute a result about spheres. And the answer is that I certainly tried to derive higher-dimensional spherical coordinates. I was able to derive the correct coordinates for dimensions 4 and 5. However, I was unable to come up with a systematic way of determining how the new angles I introduced were parameterized.
My naïve attempts to answer these questions gave me nonsense results for the volumes, so I had to find another way. Use spherical coordinates to find the volume of the triple integral, where ??? We first want to find the volume of the balloon.
If we look at the top part and the bottom part of the balloon separately, we see that they are geometric solids with known volume formulas. However, it is still worthwhile to set up and evaluate the integrals we would need to find the volume. If we calculate the volume using integration, we can use the known volume formulas to check our answers.
This will help ensure that we have the integrals set up correctly for the later, more complicated stages of the project. Express the mass \(m\) of the solid as a triple integral in spherical coordinates. In more advanced treatises—also American—on spherical functions the old convention remains in use, see e.g. 18., a triple integral in cylindrical coordinates is given.
We will set up the four triple integrals needed to find the center of mass (i.e., to compute M, Myz, Mxz and Mxy) and leave it to the reader to evaluate each integral. Because of symmetry, we expect the x- and y- coordinates of the center of mass to be 0. Cylindrical coordinates are useful when describing certain domains in space, allowing us to evaluate triple integrals over these domains more easily than if we used rectangular coordinates. Unfortunately, the convention for the notation of spherical coordinates is not standardized across disciplines. For example, in physics, the roles of $\theta$ and $\phi$ are typically reversed.
In order to correctly understand someone's use of spherical coordinates, you must first determine what notational convention this are using. You cannot assume they follow the convention used here. I'm still toying with the idea of making a construction in rectangular coordinates so that one can relate the differentials in just the right way, like the simple area of a circle method. Nonetheless, this does seem more sensible using spherical coordinates. Two important partial differential equations that arise in many physical problems, Laplace's equation and the Helmholtz equation, allow a separation of variables in spherical coordinates. The angular portions of the solutions to such equations take the form of spherical harmonics.
Use the conversion formulas to write the equations of the sphere and cone in spherical coordinates. How to perform a triple integral when your function and bounds are expressed in spherical coordinates. Use a CAS to graph the solid whose volume is given by the iterated integral in spherical coordinates as ∫02π∫3π/4π/4∫01ρ2sinφdρdφdθ. Use a CAS to graph the solid whose volume is given by the iterated integral in spherical coordinates ∫π/2π∫5π/6π/6∫02ρ2sinφdρdφdθ. Express the mass \(m\) of the solid as a triple integral in cylindrical coordinates. Express the mass \(M\) of the solid as a triple integral, with limits, in cylindrical coordinates.
Evaluate the volume of a circular cylinder of radius \(a\) and height \(h\) by means of an integral in spherical coordinates. While the following proof may not be trivial, it is worth considering how complex it might be in Cartesian coordinates. A sphere is a geometrical object in three-dimensional space that resembles the surface of a ball. Similar to a circle in two-dimensional space, a sphere can be mathematically defined as the set of all points that are at the same distance from a given point. This given point is called the center of the sphere. The distance between the center and any point on the surface of the sphere is called the radius, represented by r.
Just as we did with polar coordinates in two dimensions, we can compute a Jacobian for any change of coordinates in three dimensions. We will focus on cylindrical and spherical coordinate systems. That subscript on the r with the "perpendicular" symbol is very important. The distance r_ [/itex] is the perpendicular distance of the mass dm at (r,\theta,\phi) [/itex] from the axis of rotation, NOT its distance from the centre of the sphere, r.
In other words, r_ [/itex] is the radius of the latitude circle being traced out by the point at (r,\theta,\phi) [/itex] as the sphere rotates. If you draw a picture, you'll see that r_ [/itex] is equal to r \sin \theta [/itex], giving you an extra sine squared factor in the theta integral. That was the point...to post a full and correct derivation. Interesting derivation, I never thought about doing it this way. I use cylindrical coordinates; it eliminates the sin(\theta)[/tex] factor, but introduces a \sqrt[/tex] in one of the limits of integration for the z variable. The role of θ and φ in spherical coordinates differs between mathematicians and physicists.
When reading about physics in spherical coordinates, be careful to note how that particular author uses these variables and recognize that these identities may be rearranged. After rectangular coordinates, the two most common an useful coordinate systems in 3 dimensions are cylindrical coordinates and spherical coordinates . The coordinate $\rho$ is the distance from $P$ to the origin. If the point $Q$ is the projection of $P$ to the $xy$-plane, then $\theta$ is the angle between the positive $x$-axis and the line segment from the origin to $Q$. Lastly, $\phi$ is the angle between the positive $z$-axis and the line segment from the origin to $P$. Using spherical polar coordinates, the area element on the surface of a sphere is given by .
In this blog, I used polar coordinates to derive the well-known expression for the area of a circle, . In today's blog, I will go from 2 to 3-dimensions to derive the expression for the surface area of a sphere, which is . To do this, we need to use the 3-dimensional equivalent of polar coordinates, which are called spherical polar coordinates. As with the other multiple integrals we have examined, all the properties work similarly for a triple integral in the spherical coordinate system, and so do the iterated integrals. In order to find limits of integration for the triple integral, we'll say that ??? To convert from Cartesian coordinates, we use the same projection and read off the expressions for the spherical coordinates.
This uses the atan2 function to handle the different quadrants for $\theta$. The coordinate system is called spherical coordinates. For the following exercises, the equation of a surface in rectangular coordinates is given.
Find the equation of the surface in spherical coordinates. For the following exercises, the equation of a surface in spherical coordinates is given. Find the equation of the surface in rectangular coordinates.
36., a solid is described along with its density function. Find the mass of the solid using spherical coordinates. Approximating the volume of a standard region in space using spherical coordinates.
Convert the rectangular point (2,-2,1) to spherical coordinates, and convert the spherical point (6,π/3,π/2) to rectangular and cylindrical coordinates. Evaluate a triple integral by changing to spherical coordinates. In the previous section we looked at doing integrals in terms of cylindrical coordinates and we now need to take a quick look at doing integrals in terms of spherical coordinates. (Refer to Cylindrical and Spherical Coordinates for a review.) Spherical coordinates are useful for triple integrals over regions that are symmetric with respect to the origin. Longitude is measured as the angular distance in the closest direction from the prime meridian, or, alternatively, as a positive angle to the east and negative to the west.
Longitude is always within the range −180° to +180°. A pipeline is a cylinder, so cylindrical coordinates would be best the best choice. In this case, however, we would likely choose to orient our z-axis with the center axis of the pipeline. The x-axis could be chosen to point straight downward or to some other logical direction. The origin should be chosen based on the problem statement.



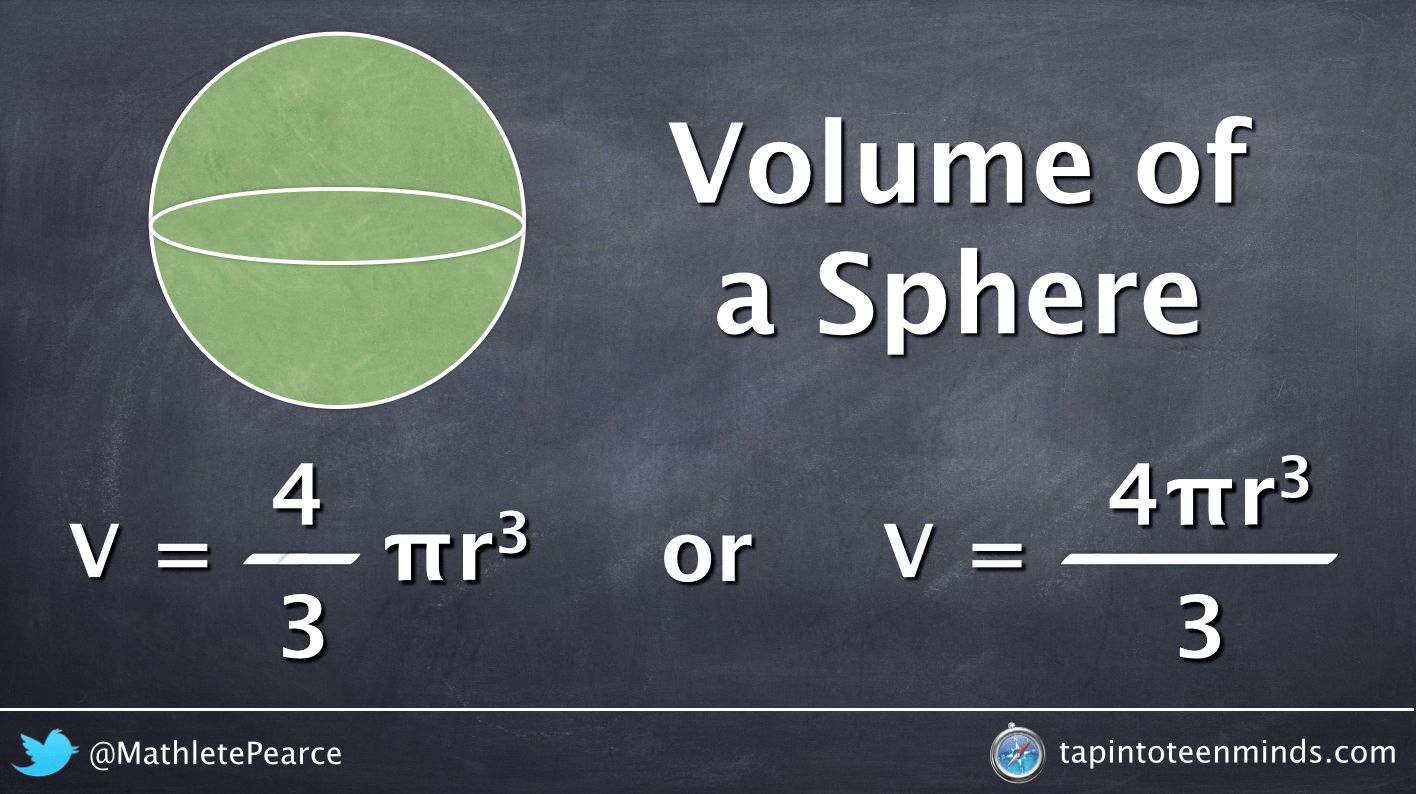





















No comments:
Post a Comment
Note: Only a member of this blog may post a comment.